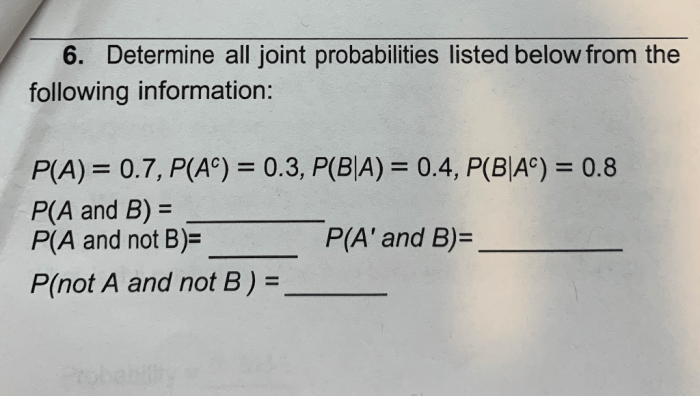
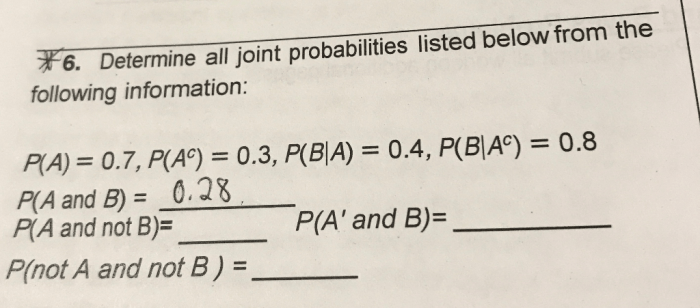
Determine all joint probabilities listed below from the following information – Joint probabilities play a crucial role in data analysis, providing insights into the likelihood of multiple events occurring simultaneously. This article delves into the concept of joint probabilities, exploring their significance, calculation methods, and applications.
Understanding joint probabilities enables researchers and analysts to make informed decisions based on the likelihood of specific outcomes. By considering the joint probabilities of different scenarios, they can assess the potential risks and benefits associated with various choices.
Joint Probabilities: Determine All Joint Probabilities Listed Below From The Following Information
Joint probabilities are crucial in data analysis, providing insights into the relationships between multiple random variables. They represent the probability of two or more events occurring simultaneously. Understanding joint probabilities is essential for accurately modeling and predicting outcomes in various fields.
Formula for Calculating Joint Probabilities
The formula for calculating the joint probability of events A and B is:
P(A ∩ B) = P(A) × P(B|A)
where P(A ∩ B) is the joint probability of events A and B, P(A) is the probability of event A, and P(B|A) is the conditional probability of event B given that event A has occurred.
Methods for Determining Joint Probabilities, Determine all joint probabilities listed below from the following information
Joint probabilities can be determined using various methods, including:
- Frequency tables:Counting the occurrences of events in a data set to estimate joint probabilities.
- Probability distributions:Using known probability distributions to model the behavior of random variables and calculate joint probabilities.
- Conditional probabilities:Applying the formula P(A ∩ B) = P(A) × P(B|A) to calculate joint probabilities.
Expert Answers
What are the key applications of joint probabilities?
Joint probabilities find applications in various fields, including finance, engineering, healthcare, and social sciences. They are used in risk assessment, reliability analysis, medical diagnosis, and many other areas.
What are the advantages of using frequency tables to determine joint probabilities?
Frequency tables provide a straightforward and intuitive method for calculating joint probabilities. They are particularly useful when dealing with discrete data and small sample sizes.
What are the limitations of using joint probabilities?
Joint probabilities can be challenging to calculate for complex events or when the number of variables involved is large. Additionally, they may not always accurately represent the real-world relationships between events.